Firemath - The MathML Editor

Example: Roots and Exponents

You might want to begin with getting acquainted with the Firemath user interface. The concepts page summarizes the different panels. During this tutorial, we refer to the concepts page whenever one of these panels is mentioned.
For our example you should start with localizing the tabs panel. Select the tab with the Greek symbols . The first thing you will notice is that you have to decide if elements are to be inserted as identifiers, operators, functions or text. See the FAQ for further details.
Make sure the identifier radio button is selected and click onto the button with the $\beta$ character. The button containing the equal sign is located in the common elements panel. Click onto this button and insert another $\beta$. Your equation should so far look like

Navigation

At this stage you should get acquainted with the possibilities to navigate through your equation. The cursor position can be changed either with the cursor buttons or with the keyboard. You can also place the cursor on a particular element by clicking onto that element.
Also, you should understand the differences between editing and presentation mode. Since the release of Firemath 0.3 your equation is shown simultaneously in both modes. In editing mode several additional elements — like pink dots — can pollute your equation. These help with the navigation and show you, where other elements can be inserted.

Attributes

You might have noticed, that the two $\beta$s in our equation are rendered italic. Firefox applies rendering rules according to which single-character identifiers are drawn italic. Here, we want the two variables to be drawn non-italic, and, for didactical reasons, we will use two different approaches to set the mathvariants to normal.
Move the cursor to the very left, such that it is located on the $\beta$. Now, we can enforce normal rendering by clicking onto the normal button in the attributes panel. For the other $\beta$ move the cursor right, until it covers the variable. A popup window appears after a click onto the attributes button . Open the menu right of 'Mathvariant', select 'normal' and click OK.

Exponents

In the next steps we will add the exponents to the variables. Since the cursor is still located over the $\beta$ on the right, we will first square this identifier. This can most easily be obtained using the corresponding button 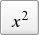in the common modifications panel. For the exponent of the other $\beta$ variable move the cursor to the very left and click onto the sub- superscript button . Notice the appearance of pink dots, indicating that the element was prepared for the addition of sub- and superscripts. Move the cursor to the dot located on the right top of the $\beta$. Exponent '6' can be inserted there in two different ways. Either click onto the corresponding button in the common identifiers panel, or insert a '6' into the text field and click onto Identifier. After this, your equation should look like

Root

What is left is to add the root function to the expression on the left. For this, move the cursor to the left until it covers the $\beta$ and its sub- and superscripts. Now, keep CTRL pressed when clicking onto the root button 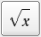in the common elements panel. Finally, place the cursor over the dot on the left top of the root function. Inserting a '3' there, gives our final result:

Before closing this unit, you might want to obtain more insight into navigation. Notice — when stepping through the equation — how the cursor either covers single or composed elements. We need the latter each time we want to change attributes of complex objects like roots, tables or elements with overscripts.